I.
Purpose:
A. The purpose of this
lab is to use the properties of right triangles to find the height of the
catwalk.
II.
Equipment:
A. 2 meter sticks
B. 1 protractor
C. Tape
D. String
E. Rubber stopper
III.
Theory:
A. This lab applies the properties of right triangles to allow the height of objects to be calculated when they are normally unable to be measured. In a right triangle you only need to know the measure of one angle and one side to be able to solve for the rest. In this lab the base is measured out in meters perpendicular to the corner of the catwalk. The device constructed from the meter stick and protractor must then find the angle. Since it is impossible to look up the end of the meter stick when it is placed directly on the ground it is done from one meter up. When the meter stick is lined up with the top of the catwalk gravity causes the rubber stopper to stay pointing towards the ground and the string is lined up with a different measurement on the protractor. The angle of is the displacement of the
measurement
read and 90o. The tangent
property can then be used to calculate
![]()
the
measure of the height since: .
To allow for human error you take measurements at more than one distance
and take the average of the calculations.
This method should return a value close to the real height as long as
there were no errors in your measurements or calculations.
IV.
Procedure:
A. Attach protractor to the end of a meter stick
B. Attach rubber stopper to meter stick by string so that it
lines up with 90o when held horizontal.
C. Mark distances from 5, 10, 15, and 20 meters away from the
catwalk.
D. Set another meter stick on end on one of the marked
distances.
E. Set end of the meter stick with the protractor on top of
the other meter stick and line it up with the top of the catwalk.
F. Subtract the reading on the protractor from 90o
and record it.
G. Repeat steps D-F two more times.
H. Use the tangent property of right triangles to calculate
the height of the catwalk from the three distances.
I.
Find the average of these
three calculations and record it.
J. Compare your Calculation to the Known height.
K. Calculate the absolute error by taking the absolute value
of the difference of your height and the known.
L. Find the Percentage of error by dividing the absolute error
by the known and multiplying by 100.
V.
Data:
Distance Away
|
Reading on Protractor |
Displacement
|
|
5m |
53 o |
37 o |
|
10m |
65 o |
25 o |
|
20m |
75o |
15 o |
VI.
Sample Calculations:
A. Calculation of height at different distances (in meters):
1. Height = distance x (tan displacement) + 1
2. H=5 x tan 37 o + 1
3. H=5 x .7536 + 1
4. H=3.768 + 1
5. H=4.768
B. 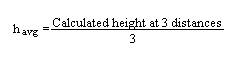 Calculation
of average height (in meters):
Calculation
of average height (in meters):
1.
![]()
2.
3. havg = 5.596
VII.
Results:
A. Calculated heights at different distances:
1. 5m = 4.768m
2. 10m = 5.663m
3. 20m = 6.358m
B. Height of Catwalk:
1. Calculated = 5.596m
2. Known = 7.960m
VIII.
Error Analysis:
A. Absolute Error:
1. 2.364m
B. Relative Percentage of Error:
1. 29.7%
C. Reasons for error:
1. Position of the protractor may have shifted.
2. The string holding the rubber stopper might have moved.
3. The meter stick may not have been held steady enough.
IX.
Conclusion
A. The height of the catwalk obtained by calculation was close but it was still off quite a bit. This inaccuracy could have been caused be several different things. Inaccuracies may have occurred due to the inaccuracy of the equipment used.